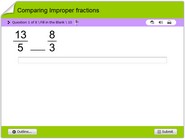
Comparing improper fractions quiz
Learn Comparing improper fractions exercise. Online math practice check your skills.
Learn how to compare improper fractions in an online quiz game
Learn how to compare improper fractions in an online quiz game. Improper fractions have numerators that are larger than their denominators. A good way of comparing them is to first reduce them to their lowest terms. This math activity is a good test for children in 4th, 5th, 6th and 7th grades. Comparison of fractions also entail using phrases like less than, greater than and equal to. Learn how to use this vocabulary in real problems. Cool math quiz online for children.
When we compare improper fractions, we are determining which one is greater or smaller than the other. An improper fraction is a fraction where the numerator (the top number) is larger than the denominator (the bottom number).
To compare improper fractions, we have a few methods, one of them is by looking at the numerators (the top numbers) and comparing them. If the numerator of one fraction is larger than the numerator of the other fraction, then the first fraction is larger. for example, 5/3 is larger than 4/3 because 5 is greater than 4.
Another way is by converting them to mixed numbers and then comparing the whole numbers. To convert an improper fraction to a mixed number, we divide the numerator by the denominator and add the remainder as a whole number. For example: 7/4 is smaller than 9/5, because when we convert 7/4 to a mixed number it will be 1 3/4 and 9/5 will be 1 4/5. 1 4/5 is bigger than 1 3/4
Another way is by cross-multiplying the fractions. This method compares the products of the numerators and denominators. For example: 7/4 is smaller than 9/5 because when we cross-multiply we get 35/4 and 36/5. and 36/5 is greater than 35/4
It’s also very important to simplify the fractions if they can be simplified before comparing them.
In conclusion, comparing improper fractions can be done by comparing the numerators, converting them to mixed numbers, or cross-multiplying them. The most important thing to remember is that the fractions need to have the same denominator to be able to compare them directly. It’s also a good idea to practice comparing different improper fractions to become comfortable and confident with the process.