Addition of fractions with large denominators quiz

Learn how to do Addition of fractions with large denominators through this online quiz

 

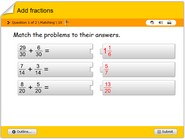
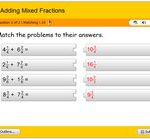
Math quiz involving addition of fractions with large denominators

Math quiz involving addition of fractions with large denominators for children in 4th, 5th, 6th and 7th grades. This is an interactive online math test which kids can use to test their skills after school. In each exercise, children have to solve an addition problem and find the corresponding answer. After doing this, players should drag and match to appropriate locations. This quiz could also be used as a math game or as a multiple choice question trivia. Learn math with fun.

When we add fractions, we’re finding the total amount when we combine two or more parts together. One way we can add fractions is by making sure that the denominators, or bottom numbers, are the same.

However, sometimes the denominators can be very large numbers, making it difficult to add the fractions together. In these cases, we can use a method called “finding a common denominator” to help us add the fractions.

A common denominator is a number that both fractions can be converted to, so that they have the same denominator. To find a common denominator for two fractions, we can use the least common multiple (LCM) of the denominators. The least common multiple is the smallest number that both denominators can divide into without leaving a remainder.

For example, let’s say we want to add the fractions 2/15 and 3/20. To find a common denominator, we can use the least common multiple of 15 and 20. The least common multiple of 15 and 20 is 60. This means that we can convert 2/15 to 8/60 and 3/20 to 9/60, so that both fractions have the same denominator of 60.

Now that we have a common denominator, we can add the fractions together: 8/60 + 9/60 = 17/60

In this case, 17/60 is a simplified fraction, we can use the greatest common factor (GCF) to simplify more. The GCF of 17 and 60 is 1.

Another way of achieving the same result is by multiplying the numerator and denominator of the first fraction by the same value, and then doing the same for the second fraction.

For example, you could multiply the numerator and denominator of the first fraction by 4 and the numerator and denominator of the second fraction by 3, this way: (2/15) * (4/4) = 8/60 and (3/20) * (3/3) = 9/60, then add them 8/60 + 9/60 = 17/60

It’s important to note that when working with fractions with large denominators, it is important to simplify the fractions to lowest terms whenever possible, as we showed in the previous example.

Another example let’s say we have to add 2/45 + 5/90, to add them we need to find a common denominator. We can use the LCM of 45 and 90, which is 90. Then we convert the fractions into 9/90 and 5/90, which we can add them together to get 14/90

It’s important to note that sometimes, finding a common denominator for fractions with large denominators can be a bit more difficult and time consuming, but by breaking it down and following these steps, it can be a manageable task.

In summary, when adding fractions with large denominators, it’s necessary to find a common denominator. The least common multiple of the denominators is usually the common denominator. To convert the fractions, we can multiply both the numerator and denominator by the same value. And remember it’s always a good idea to simplify the fractions to lowest terms whenever possible.